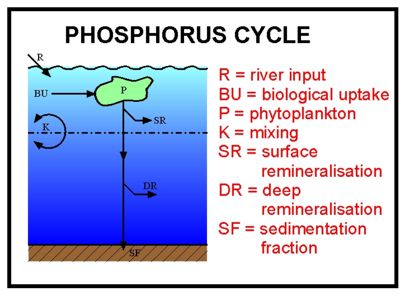
The article provides answers to the questions posed in the phosphorus model worksheet.
Worksheet answers
1. Try some different types of initial conditions: e.g. (a) halved deep phosphate, all else the same; (b) doubled deep P, all else the same; (c) ten-fold higher surface phytoplankton, surface and deep P the same. Does the model converge to a stable equilibrium (= control phosphorus) in each case? [remember to reset initial conditions before making each change, so previous changes are wiped clear]
The model will (should!) converge in all cases, for absolutely any initial conditions.
2. Is the final state always the same?
Yes, final steady state should always be the same (if run for long enough – there may be minor differences because equilibrium hasn’t quite been reached).
3. The return to the equilibrium state is governed by the balance between inputs and outputs. Examine the size of the burial flux: (a) immediately after doubling of deep phosphate, all else the same; and (b) after halving of deep phosphate. Does the behaviour of the burial flux in these situations help the model return to equilibrium?
Yes, burial flux is larger after doubling deep PO4, smaller after halving it.
4. Try adding 1.0 mmol PO4 m-3 to: (a) the surface box, and (b) the deep box. Which has the greater effect on phytoplankton concentrations over the next few thousand years? Why?
Increase of deep phosphate concentration has a greater impact. This is because the deep box has a greater depth and therefore a greater volume. Increasing the deep box concentration by 1 mmol m-3 therefore adds more P to the system as a whole.
5. What impact does the extra phosphate have on the biology? What impact does the induced biological change have on the extra phosphate?
More PO4 → more PO4 in upwelled/mixed up water → more phytoplankton → more export → more burial → increased rate of loss of P from the system → PO4 levels come back down again.
6. Reset to the default initial conditions and then examine the effects of altering RP, the river supply of phosphate. What are the effects on phytoplankton concentrations and total primary production (TPP) of: (a) doubling RP, and (b) halving RP?
Doubling RP doubles surface box phytoplankton concentration and TPP. Halving RP halves them both.
7. How do the steady-state surface phosphate concentrations compare, between the two runs? How can you square this with the effects on the biology?
Steady-state surface phosphate is identical in the two runs. The important point here is that it is nutrient supply not instantaneous nutrient concentration that controls productivity. This has been an important misconception in the past.
8. Using the default value of RP, now try altering the value of K (intensity of ocean mixing/overturning) by doubling its value. Wally Broecker & Tsung-Hung Peng (Tracers in the Sea) calculated in 1982 (no computer models back then) that this change in K should lead to the deep box phosphate concentration beginning a long, slow decline towards a new, lower steady-state value. They calculated (their figure 6.2) that it should take about 35,000 years for half of the change to be brought about. Do you agree? [to calculate this, use the facility to "save data" and then examine it in Excel or Matlab]
Doubling K does produce a long slow decline in deep phosphate concentration. The timescales are different. In this model half of the change is achieved in about 9300 years, rather quicker than estimated by Broecker & Peng.
Other related pages
Phosphorus model overview
Phosphorus model details
Phosphorus model model cons
Phosphorus model pros
Phosphorus model help
Phosphorus model worksheet
References
Broecker, W.S. & Peng, T.H. (1982) Tracers in the Sea, Eldigio Press, pp. 275-284.
External links
Description of the chemical element phosphorus.
Description of the phosphorus cycle.